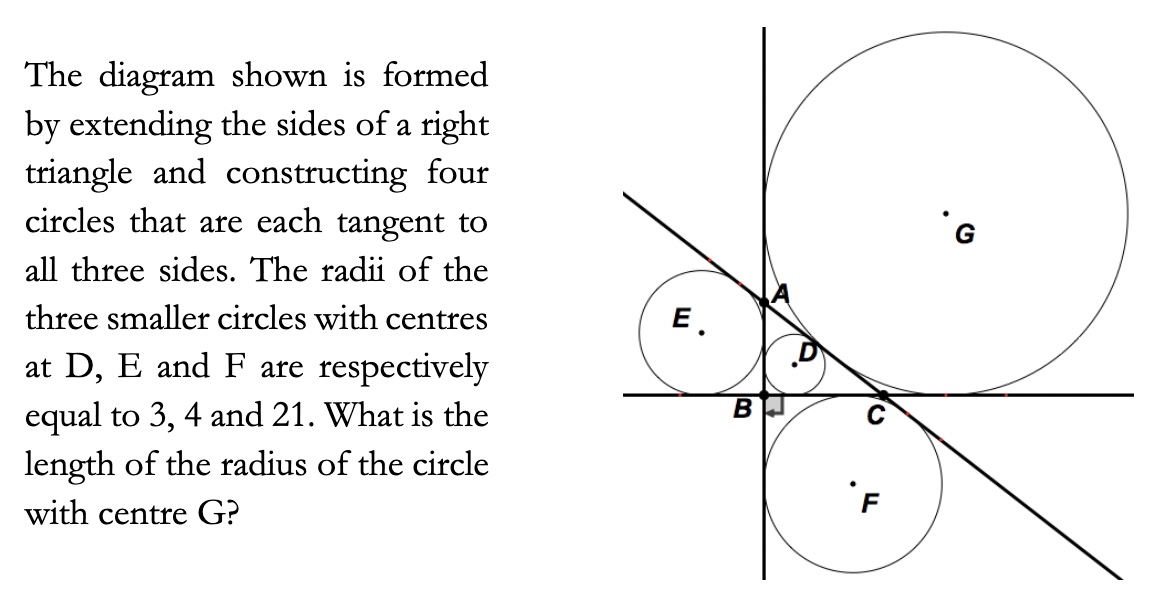
In Round 2 of the South African Mathematics Olympiad 2016, the following problem was posed.

Challenge
1) First try and solve the above problem yourself before continuing below.
Explore More
Interestingly the problem is actually a special case of a theorem related to any right triangle.
2) Click on the 'Show Radii of Circles' button.
3) Drag any of A, B or C and observe how the radii change dynamically. Can you see a relationship between the four radii?
4) Click on the 'Show Sum of Radii' button.
5) What do you notice? Is it always true? Make a conjecture and explore by dragging any of the red vertices.
Generalization of SA Mathematics Olympiad 2016 Problem R2 Q20
Challenge
6) Can you explain why (prove) your observation in 5) is true?
7) Can you prove the result in more than one way?
Published Paper
Read my paper A Multiple Solution Task: Another SA Mathematics Olympiad Problem in Learning and Teaching Mathematics, No. 22, 2017, pp. 42-46 about the problem above.
Further Generalization
It is natural to ask if the same relationship generalizes to any triangle, and if not, if there is another more general relationship relating the four radii to each other?
8) Use your own dynamic software to make constructions and to explore this for yourself.
Check your findings in 8) at this Wolfram MathWorld link: Exradius.
Related Links
SA Mathematics Olympiad Problem 2016, Round 1, Question 20
SA Mathematics Olympiad 2022, Round 2, Q25
An extension of the IMO 2014 Problem 4
A 1999 British Mathematics Olympiad Problem and its dual
Dirk Laurie Tribute Problem
Extangential Quadrilateral
Triangulated Tangential Hexagon theorem
Theorem of Gusić & Mladinić
Conway's Circle Theorem as special case of Side Divider (Windscreen Wiper) Theorem
Japanese Circumscribed Quadrilateral Theorem
External Link
SA Mathematics Olympiad
Questions and worked solutions for past South African Mathematics Olympiad papers can be found at this link.
(Note, however, that prospective users will need to register and log in to be able to view past papers and solutions.)
***************
Free Download of Geometer's Sketchpad
Back to "Dynamic Geometry Sketches"
Back to "Student Explorations"
First created in 2016 with JavaSketchpad by Michael de Villiers, updated to WebSketchpad 28 Sept 2024.